Triangulation of $\Bbb{S}^{n}$

Triangulation of $\Bbb{S}^{2}$

$\Bbb{S}^{2}$ can be triangulated into eight triangular regions. This triangulation has the same symmetry group as the octahedron. The triangulation basically consists of connecting the six points $\left(\pm 1,0,0\right)$, $\left(0, \pm 1, 0\right)$, $\left(0, 0, \pm 1\right)$ with great circles in the x-y, y-z and z-x planes. Cutting the sphere along these lines, and then laying out the eight resulting triangles in a plane can be thought of as another visualization technique for two-dimensional beings.

$\downarrow$

Triangulation of $\Bbb{S}^{3}$

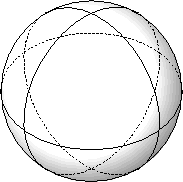
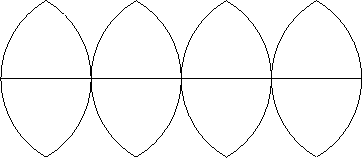
Similarly, $\Bbb{S}^{3}$ can be triangulated into sixteen tetrahedral regions by connecting the eight points $\left( \pm 1, 0, 0, 0\right)$, $\left( 0, \pm 1, 0, 0\right)$, $\left( 0, 0, \pm 1, 0,\right)$, $\left( 0, 0, 0, \pm 1\right)$ with great spheres. This triangulation has the same symmetry as the sixteen-cell polytope. Like the triangulation of $\Bbb{S}^{2}$, this can be used as another method of visualization of $\Bbb{S}^{3}$, and it will be, later.

$\downarrow$

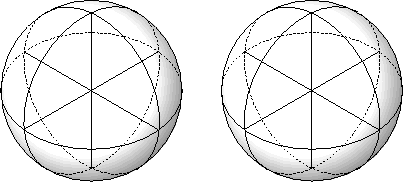
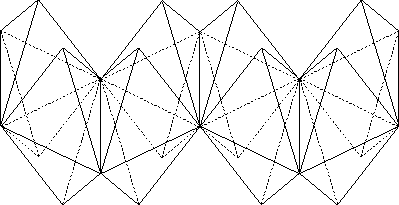
Notice that there are a great many more possibilities for how these sixteen tetrahedra can be arranged than there are for the triangulation of $\Bbb{S}^{2}$. Might there be a particularly orderly arrangement for them, that had no analogue in $\Bbb{S}^{2}$?

Triangulation of $\Bbb{S}^{n}$

Since an analogue of the symmetry group of the octahedron and the sixteen-cell exists in every dimension, we can generalize this process of triangulation to $\Bbb{S}^{n}$, decomposing it into $2^{n+1}$ n-cells.